1979 USAMO Problems/Problem 2

Problem

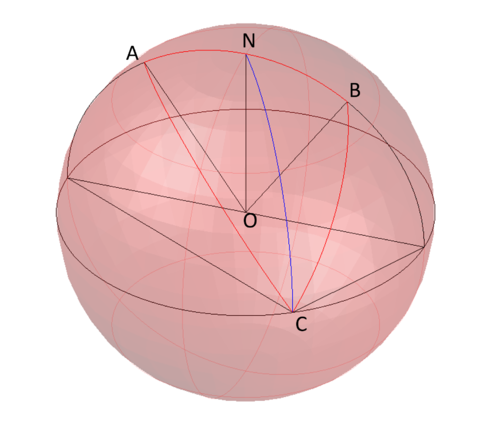
$N$ is the north pole. $A$ and $B$ are points on a great circle through $N$ equidistant from $N$ . $C$ is a point on the equator. Show that the great circle through $C$ and $N$ bisects the angle $ACB$ in the spherical triangle $ABC$ (a spherical triangle has great circle arcs as sides).

Solution

Since $N$ is the north pole, we define the Earth with a sphere of radius one in space with $N=(0,0,1)$ and sphere center $O=(0,0,0)$ We then pick point $N$ on the sphere and define the $xz$ -plane as the plane that contains great circle points $A$ , $B$ , and $N$ with the $x$ -axis perpendicular to the $z$ -axis and in the direction of $A$ .

Using this coordinate system and $x$ , $y$ , and $z$ axes $A=(cos(\phi),0,sin(\phi))$ where $\phi$ is the angle from the $xy$ -plane to $A$ or latitude on this sphere with $\frac{-\pi}{2} < \phi < \frac{\pi}{2}$

Since $A$ and $B$ are points on a great circle through $N$ equidistant from $N$ , then $B=(-cos(\phi),0,sin(\phi))$

Since $C$ is a point on the equator, then $C=(cos(\theta),sin(\theta),0)$ where $\theta$ is the angle on the $xy$ -plane from the origin to $C$ or longitude on this sphere with $-\pi < \phi \le \pi$

We note that vectors from the origin to points $N$ , $A$ , $B$ , and $C$ are all unit vectors because all those points are on the unit sphere.

So, we're going to define points $N$ , $A$ , $B$ , and $C$ as unit vectors with their coordinates.

We also define the following vectors as follows:

Vector $\overrightarrow{V_{CN}}$ is the unit vector in the direction of arc $CN$ and tangent to the great circle of $CN$ at $C$

Vector $\overrightarrow{V_{CA}}$ is the unit vector in the direction of arc $CA$ and tangent to the great circle of $CA$ at $C$

Vector $\overrightarrow{V_{CB}}$ is the unit vector in the direction of arc $CB$ and tangent to the great circle of $CB$ at $C$

To calculate each of these vectors we shall use the cross product as follows:

$\overrightarrow{V_{CN}}=(\overrightarrow{C}\times\overrightarrow{N})\times\overrightarrow{C}$

$\overrightarrow{V_{CN}}=(\left\langle cos(\theta),sin(\theta),0 \right\rangle\times\left\langle 0,0,1 \right\rangle)\times \left\langle cos(\theta),sin(\theta),0 \right\rangle$

$\overrightarrow{V_{CN}}=\left\langle sin(\theta),-cos(\theta),0 \right\rangle\times \left\langle cos(\theta),sin(\theta),0 \right\rangle$

$\overrightarrow{V_{CN}}=\left\langle 0,0,sin^{2}(\theta)+cos^{2}(\theta) \right\rangle$

$\overrightarrow{V_{CN}}=\left\langle 0,0,1 \right\rangle$

Vector $\overrightarrow{V_{CA}}$ :

$\overrightarrow{V_{CA}}=(\overrightarrow{C}\times\overrightarrow{A})\times\overrightarrow{C}$

$\overrightarrow{V_{CA}}=(\left\langle cos(\theta),sin(\theta),0 \right\rangle\times\left\langle cos(\phi),0,sin(\phi) \right\rangle)\times \left\langle cos(\theta),sin(\theta),0 \right\rangle$

$\overrightarrow{V_{CA}}=\left\langle sin(\theta)sin(\phi),-cos(\theta)sin(\phi),-sin(\theta)cos(\phi) \right\rangle\times \left\langle cos(\theta),sin(\theta),0 \right\rangle$

Since we're only interested in the $z$ component of the vector

$\overrightarrow{V_{CA}}=\left\langle V_{CA_{x}},V_{CA_{y}},sin^{2}(\theta)sin(\phi)+cos^{2}(\theta)sin(\phi) \right\rangle$

$\overrightarrow{V_{CA}}=\left\langle V_{CA_{x}},V_{CA_{y}},sin(\phi) \right\rangle$

Vector $\overrightarrow{V_{CB}}$ :

$\overrightarrow{V_{CB}}=(\overrightarrow{C}\times\overrightarrow{b})\times\overrightarrow{C}$

$\overrightarrow{V_{CB}}=(\left\langle cos(\theta),sin(\theta),0 \right\rangle\times\left\langle -cos(\phi),0,sin(\phi) \right\rangle)\times \left\langle cos(\theta),sin(\theta),0 \right\rangle$

$\overrightarrow{V_{CB}}=\left\langle sin(\theta)sin(\phi),-cos(\theta)sin(\phi),sin(\theta)cos(\phi) \right\rangle\times \left\langle cos(\theta),sin(\theta),0 \right\rangle$

Since we're only interested in the $z$ component of the vector

$\overrightarrow{V_{CB}}=\left\langle V_{CB_{x}},V_{CB_{y}},sin^{2}(\theta)sin(\phi)+cos^{2}(\theta)sin(\phi) \right\rangle$

$\overrightarrow{V_{CB}}=\left\langle V_{CB_{x}},V_{CB_{y}},sin(\phi) \right\rangle$

Since we're working with unit vectors, then we can use dot products on the vectors with their angles as follows:

$cos(\angle ACN) = \overrightarrow{V_{CA}}\cdot \overrightarrow{V_{CN}}$

$cos(\angle ACN) = \left\langle V_{CA_{x}},V_{CA_{y}},sin(\phi) \right\rangle \cdot \left\langle 0,0,1 \right\rangle = 0*V_{CA_{x}}+0*V_{CA_{y}}+1*sin(\phi)=sin(\phi)$

Likewise,

$cos(\angle BCN) = \overrightarrow{V_{CB}}\cdot \overrightarrow{V_{CN}}$

$cos(\angle BCN) = \left\langle V_{CB_{x}},V_{CB_{y}},sin(\phi) \right\rangle \cdot \left\langle 0,0,1 \right\rangle = 0*V_{CB_{x}}+0*V_{CB_{y}}+1*sin(\phi)=sin(\phi)$

Therefore,

$cos(\angle ACN) = cos(\angle BCN)$ and thus $\angle ACN = \angle BCN$

Since those angles are equal, it proves that the great circle through $C$ and $N$ bisects the $\angle ACB$ in the spherical triangle $ABC$

~Tomas Diaz. orders@tomasdiaz.com

Alternate solutions are always welcome. If you have a different, elegant solution to this problem, please add it to this page.

1979 USAMO (Problems • Resources)
Preceded by Problem 1	Followed by Problem 3
1 • 2 • 3 • 4 • 5
All USAMO Problems and Solutions

Page

Toolbox

Search

1979 USAMO Problems/Problem 2

Problem

Solution

See Also