2007 AIME II Problems/Problem 9
Contents
Problem
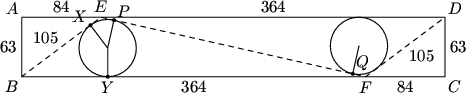
Rectangle ![]() is given with
is given with ![]() and
and ![]() Points
Points ![]() and
and ![]() lie on
lie on ![]() and
and ![]() respectively, such that
respectively, such that ![]() The inscribed circle of triangle
The inscribed circle of triangle ![]() is tangent to
is tangent to ![]() at point
at point ![]() and the inscribed circle of triangle
and the inscribed circle of triangle ![]() is tangent to
is tangent to ![]() at point
at point ![]() Find
Find ![]()
Solution
Solution 1
Several Pythagorean triples exist amongst the numbers given. ![]() . Also, the length of
. Also, the length of ![]() .
.
Use the Two Tangent Theorem on ![]() . Since both circles are inscribed in congruent triangles, they are congruent; therefore,
. Since both circles are inscribed in congruent triangles, they are congruent; therefore, ![]() . By the Two Tangent theorem, note that
. By the Two Tangent theorem, note that ![]() , making
, making ![]() . Also,
. Also, ![]() .
. ![]() .
.
Finally, ![]() . Also,
. Also, ![]() . Equating, we see that
. Equating, we see that ![]() , so
, so ![]() .
.
Solution 2
By the Two Tangent Theorem, we have that ![]() . Solve for
. Solve for ![]() . Also,
. Also, ![]() , so
, so ![]() . Since
. Since ![]() , this can become
, this can become ![]()
![]() . Substituting in their values, the answer is
. Substituting in their values, the answer is ![]() .
.
Solution 3
Call the incenter of ![]()
![]() and the incenter of
and the incenter of ![]()
![]() . Draw triangles
. Draw triangles ![]() .
.
Drawing ![]() , We find that
, We find that ![]() . Applying the same thing for
. Applying the same thing for ![]() , we find that
, we find that ![]() as well. Draw a line through
as well. Draw a line through ![]() parallel to the sides of the rectangle, to intersect the opposite side at
parallel to the sides of the rectangle, to intersect the opposite side at ![]() respectively. Drawing
respectively. Drawing ![]() and
and ![]() , we can find that
, we can find that ![]() . We then use Heron's formula to get:
. We then use Heron's formula to get:
![]() .
.
So the inradius of the triangle-type things is ![]() .
.
Now, we just have to find ![]() , which can be done with simple subtraction, and then we can use the Pythagorean Theorem to find
, which can be done with simple subtraction, and then we can use the Pythagorean Theorem to find ![]() .
.
Solution 4
Why not first divide everything by its greatest common factor, ![]() ? Then we're left with much simpler numbers which saves a lot of time. In the end, we will multiply by
? Then we're left with much simpler numbers which saves a lot of time. In the end, we will multiply by ![]() .
.
From there, we draw the same diagram as above (with smaller numbers). We soon find that the longest side of both triangles is 52 (64 - 12). That means:
![]() indicating
indicating ![]() so
so ![]() .
.
Now, we can start applying the equivalent tangents. Calling them ![]() ,
, ![]() , and
, and ![]() (with
(with ![]() being the longest and a being the shortest),
being the longest and a being the shortest),
![]() is the semi perimeter or
is the semi perimeter or ![]() . And since the longest side (which has
. And since the longest side (which has ![]() ) is
) is ![]() ,
, ![]() .
.
Note that the distance ![]() we desired to find is just
we desired to find is just ![]() . What is
. What is ![]() then?
then? ![]() . And
. And ![]() is
is ![]() . Therefore the answer is
. Therefore the answer is ![]() ...
... ![]()
Multiply by ![]() back again (I hope you remembered to write this in
back again (I hope you remembered to write this in ![]() letters on top of the scrap paper!), we actually get
letters on top of the scrap paper!), we actually get ![]() .
.
Solution 5
Scaling everything by 7, we have that ![]() . Note that if the perpendicular of
. Note that if the perpendicular of ![]() dropped down to
dropped down to ![]() is
is ![]() , then
, then ![]() . But
. But ![]() and so we have a
and so we have a ![]() right triangle with
right triangle with ![]() meaning
meaning ![]() . Now, by symmetry, we know that
. Now, by symmetry, we know that ![]() meaning
meaning ![]() . If the tangent of the circle inscribed in
. If the tangent of the circle inscribed in ![]() is tangent to
is tangent to ![]() at
at ![]() , then if
, then if ![]() we have a system of equations.
we have a system of equations. ![]() . We can then solve for
. We can then solve for ![]() , and since
, and since ![]() , the rest follows.
, the rest follows.
See also
| 2007 AIME II (Problems • Answer Key • Resources) | ||
| Preceded by Problem 8 |
Followed by Problem 10 | |
| 1 • 2 • 3 • 4 • 5 • 6 • 7 • 8 • 9 • 10 • 11 • 12 • 13 • 14 • 15 | ||
| All AIME Problems and Solutions | ||
The problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.